Knowing exactly how steep a slope is can be important information for understanding potential avalanche danger. Or, even if you’re not concerning yourself for the sake of safety, but instead, to prop up your ego, having an accurate measurement is good to have in either case.
The best way to do that is with a clinometer (often referred to as an inclinometer), a device to measure the incline of the slope. There are several options available in the new millenium. The classic clinometer is a ball bearing suspended in a cylinder of oil, bent into an arc with marks every degree. Any decent snow study kit comes with one.
For a time Ortovox’s S1 avalanche transceiver could measure slope angle and, of course, there is a smart phone app for that as well. My fave is Pieps electronic 30°+ inclinometer that straps to a ski pole. It doesn’t double as a phone and can’t surf the internet, it just indicates what angle it is oriented at and also senses the air temperature (C°/F°). It is fast and accurate (±1°), but two decimal points more expensive than a phone app.
Although these higher tech options are certainly nice to have, when you’re going light, or you’re on a tight budget there is another, less precise but sufficiently accurate way to estimate slope angles. All it takes is a pair of ski poles and a cursory understanding of basic trigonometry. Incidentally, K2’s LockJaw® ski poles come with marks on the shafts to help with this, and their Carbon/Carbon model also has a bubble clinometer under the handle.
Now you don’t need to remember all the trigonometric definitions for sine, cosine, secant, cosecant, tangent and cotangent, or bring a calculator along either. You only need to know what the tangent of a few key angles are and you’ll be set.
Trigonometry Refresher
for Ski Mountaineers
First, a little high school math refresher.
If you construct a right triangle (a triangle with a right angle (90°) in one of the corners), the following equation will be true:
tanθ = Rise ÷ Run
-OR- more commonly shown as:
tanθ = Y / X
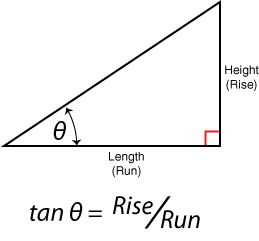
If you construct a right triangle — a triangle with a right (90°) angle in one of the corners — the above equation will be true.
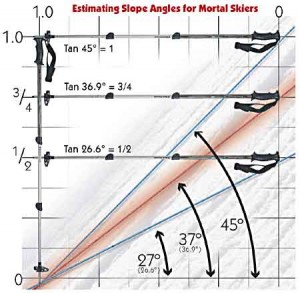
Now, if you pick some easy to remember angles, you get a table that yields only one easy to remember tangent, i.e., tan(45º) = 1
| Angle | Tangent |
| 15º | 0.267 |
| 30º | 0.577 |
| 45º | 1.0 |
| 60º | 1.73 |
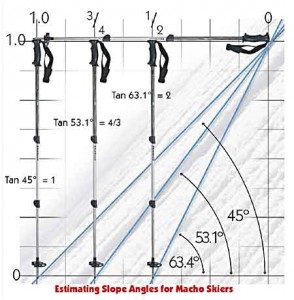
However, if you reverse priorities, and make the tangent the easy-to-remember number, or more to the point, the easy-to-approximate number, the following table results:
| Angle | Tangent | Rise | Run |
| 26.6º | ½ | 0.5 | 1.0 |
| 34.0° | 5/8 | 0.675 | 1.0 |
| 36.9° | ¾ | 0.75 | 1.0 |
| 41.2° | 7/8 | 0.875 | 1.0 |
| 45° | 1 | 1.0 | 1.0 |
| 53.10° | 4/3 | 1.0 | 0.75 |
| 56.0° | 11/8 | 1.0 | 0.675 |
| 63.4° | 3/2 | 1.0 | 0.5 |
Therefore, to measure a slope, all you need do is form a right triangle using your ski poles and the ski slope.
Start with the height pole. Make sure it is perfectly vertical (as close as you can approximate). Then cross your other pole at a 90° angle and move it down the upright pole until it touches the surface of the slope.
Then, based on the key positions shown above (Fig. 1 & 2), interpolate what the slope angle is based on the above table of key, easy to estimate positions.
This is an updated version of an article that was first published in Couloir Vol. XV-3, December 2002.
© 2013



1 comment
2 pings
Another super easy technique uses a compass and the fact that bubbles float. Rotate the compass ring so West lines up with the direction arrow, lay a ski pole on the slope of interest, and place the left edge of the compass on the pole, pointing uphill. The bubble will line up with the slope angle, accurate to +/- 2 or 3 degrees depending on the bubble size and how closely the pole angle matches the slope angle.
[…] If you want an even lighter option, consider using a your ski poles, brain and trigonometry. […]
[…] is over 25 degrees in steepness when you’re sinking past your calves while wearing just boots. See this link to estimate slope angles with your hiking poles. Often to avoid avalanche terrain, the safest place is the crest of ridgelines, but that can force […]